After 36 years of travel, the Voyager 1 spacecraft had officially been announced by NASA to have entered interstellar space, the vast void of the galaxy that separates the stars. Pioneer 10, travelling in the opposite direction to Voyager 1, is the second most distant physical object made by humans. Pionner 11 and Voyager 2 come in 3rd and 4th place respectively, as shown in the diagram below.
These spacecraft will be humanity's first physical objects that leave the solar system. Although exploration of our solar system is nowhere near completion it is clear that in less than a century of exploration we have essentially traversed the solar system using our machines.
Due to the theory of Special Relativity there is a cosmic speed limit, the speed of light.
The speed of light is a very big number by planetary standards, a beam of light in a vacuum travels 299,792,458 meters per second.
If the Earth's equator is 40,075.02 km long, something moving at light speed would make it around the equator about 7.48 times in one second. This makes it possible for a planet-wide communication system, such as the internet.
However, on a cosmic scale the speed of light seems less than adequate to traverse space, even using electronic signals. When New Horizon's reaches Pluto, it will be 5,906,376,272 km away from earth. This is 39.2 times the distance from the earth to the sun. It is common enough knowledge that it takes about 8 minutes for light to reach the earth from the sun so it takes roughly 5.2 hours for radio signals to reach earth from the probe and vice-versa. This means if we were using remote controls to steer the probe it would take 10.4 hours to perform a single command. Therefore for spacecraft to go to the planets and work in real time they must be at least semi-intelligent and robotic. The further a probe must go from earth, the more automated and intelligent it must be. Spacecraft destined for the stars and beyond would have to be of an intelligence close to, or even possibly greater than, a human to make the trip worthwhile as it would be so far away that any problems would have to be solved as they happen.
On interstellar distanced, radio signals emitted from earth, which travel at the speed of light, travel staggeringly slow in terms of the timescales at which humans are used to. As a civilization we have been only transmitting radio signals of significant strength for 8 decades, a human lifetime, long enough only to create a small radio bubble around our region of the galaxy.
Even in about another 100 years time, our radio bubble from our radio communications will be minuscule compared to the scale of the galaxy.
An illustration of what a radio bubble (yellow speck) from a civilization transmitting radio signals for 200 years looks like on a galactic scale.
On interstellar distanced, radio signals emitted from earth, which travel at the speed of light, travel staggeringly slow in terms of the timescales at which humans are used to. As a civilization we have been only transmitting radio signals of significant strength for 8 decades, a human lifetime, long enough only to create a small radio bubble around our region of the galaxy.
Even in about another 100 years time, our radio bubble from our radio communications will be minuscule compared to the scale of the galaxy.
An illustration of what a radio bubble (yellow speck) from a civilization transmitting radio signals for 200 years looks like on a galactic scale.
Special Relativity's cosmic speed limit essentially traps both humans and our technology in a time bubble when separated in a vast expanse of space. However Special Relativity is not the only physical law governing space and time. There is another aspect of the theory of relativity which is far more complicated and has many mysterious predictions and this is General Relativity which is at the core of understanding motion in a non-classical context, where the concepts of action-reaction do not appear in the same way as we would understand if we were studying from a Newtonian context alone.
General Relativity and The Mach Principle: Einstein's view of Gravity
Newton famously discovered the concept of gravity when he apparently witnessed an apple falling from a tree at the same time he saw the moon set and asked the question, "If an apple falls, does the moon also fall?". This question led to development of Newtonian mechanics, the foundation of modern physics. Moreover, Newton developed calculus in order to quantify the curvature of objects in the gravitational interaction. In essence this also showed us that we can make great progress from a mathematical concept of the physical forces without really knowing the full nature of them. Newton knew how gravity worked by his calculations but he did not now the nature of the force.This lack of the true physical concept of gravity meant that there were mysteries in our own solar system long after Newton's laws were used to predict planetary orbits. Most famously, the orbit of Mercury displayed eccentricities that could only be accounted for if another planet, called Vulcan, was tugging at it from the opposite side of the sun. Vulcan was never discovered and for a very good reason. It did not exist.
The true concept of gravity came from the idea of spacetime curvature, summarized in the Einstein Field Equation which is a result of the theory of General Relativity.
where
 is the Ricci curvature tensor,
is the Ricci curvature tensor,  the scalar curvature,
the scalar curvature, 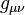 the metric tensor,
the metric tensor,  is Newton's gravitational constant and
is Newton's gravitational constant and  the stress–energy tensor.
the stress–energy tensor.According to the Einstein Equation above, matter and energy tell spacetime how to curve and in turn spacetime tells matter and energy how to move.
One of the purposes of introducing the concept of a tensors is the fact that it can represent a collection of attributes associated with some point in spacetime. As a generalisation, the Stress-Energy tensor [Tμν] can collectively describe the energy density, momentum, flux, pressure and shear stress associated with any unit volume of spacetime. The diagram below is representative of the potential scope of the stress-energy tensor:

Like the Ricci tensor, the stress-energy tensor is a rank (0,2) tensor and therefore can be expressed in the form of 4x4 matrix associated with the [t,x,y,z] components of spacetime.
- In isolation, the 3x3 matrix [T11-T33] is sometimes referred to as the stress tensor as every element corresponds to the stress, i.e. force per unit area, in the [μ] direction that acts on a surface normal to the [ν] direction.
- With reference to the table above, the suffix [1,2,3] might be loosely associated with directions [x,y,z], where [T13] is the stress acting in the [x] direction and [T33] is the stress acting on it in the [z] direction, with respect to a surface in the [xy] plane.
- The diagonal elements of this 3x3 matrix, i.e. [T11, T22, T33], are stresses that can be interpreted as pressure, i.e. force per unit area. The remaining elements are shear stresses, which for simplicity can be set to zero for the scope of most discussions.
- In contrast, the element [T00] is the energy density, i.e. energy per unit volume, at a given point in spacetime.
- While the elements [T01, T02, T03] correspond to the energy flux, e.g. [T01] is the energy flux in the [x] direction per unit volume.
- Alternatively, the element [T10, T20, T30] correspond to the momentum density through a surface normal to a given unit area per unit time.
This can initially appear to be leading towards a very complicated description of spacetime, but in the context of cosmology, spacetime is often modelled on the idea of a prefect fluid, where the complexity of the previous table reduces to:
In this case, the stress-energy tensor is defined by the matter-energy density [ρ] and pressure [P] of a unit volume of spacetime. Among other things, this can be correlated to a specific solution of Einstein field equation known as the Friedmann solution, which is more or less the basis for the entire field of cosmology:

Friedmann equation and its terms. It is useful to point out that whenever we are talking about General Relativity we are usually talking about it in the context of cosmology, hence in many ways we do not use Einstein's equations directly but the solutions of them. Newton's laws, in comparison, dominate on interplanetary and interstellar scales and even then when we are talking about Newtonian mechanics we usually use the solutions which incorporate Newton's Laws, such as Kepler's Laws.
The fundamental difference between Einsteinian and Newtonian laws for gravity is how mass density is the source of gravity in Newton's Law for gravity, whereas the energy and momentum density in spacetime is the source of gravitation in Einstein's General Relativity.
However, the Einstein equation makes a further step not only by relating spacetime curvature and the motion of a matter-energy system but by providing the implication that accelerated motion and the effects of gravity are themselves not distinguishable.
Therefore, In a local medium, acceleration motion should generate an inertial reaction force under this theory.
From Newtonian mechanics, the property of inertia is an inherent property of matter that is independent of all other things in the universe. It is unaffected by the presence or absence of the other matter elsewhere in the universe. This is one interpretation of Newton's Third Law.
General relativity theory says that the origin of inertial reaction forces in accelerated local objects( accelerated relative to the "fixed stars") creates a force indistinguishable from a gravitational "field" created here by the presence of distant matter apparently acting on the accelerating object.
This was understood under the famous, elevator thought experiment.
This was one of Einstein's largest guiding factor's for developing his theory of General Relativity in the first place and was what he referred to as the Mach Principle.
Consider the following
You are standing in a field looking at the stars. Your arms are resting freely at your side, and you see that the distant stars are not moving. Now start spinning. The stars are whirling around you and your arms are pulled away from your body. This is the centrifugal force, but why should it act when the stars are whirling but not act when you are standing still; if all reference frames are equal, then why should the change in symmetry matter?

The Mach Principle implies that this is not just a coincidence and that there is a physical law that relates the motion of the distant stars to the local inertial frame. If you see all the stars whirling around you, then there is some physical law which would cause the centrifugal force to lift your arms. Moreover it should also appear to an outside observer that you are under a centrifugal
force.
force.
Due to Jupiter's rapid rotation the effect of the centrifugal force is obvious. Earth is also an oblate spheroid, with the poles being slightly flatter than the equator.
The summary of the idea of the Mach Principle, although vague, is essentially this:
"mass out there influences inertia here and does not influence itself".
In General Relativity, spacetime is "curved", and momentum(and hence force) at a point cannot be directly meaningfully corresponded to momentum(and force) at a different point in spacetime. To correspond quantities from different points in spacetime, we need something called "parallel transport" which maps the momentum(or force) space at one point to the momentum(or force) space at another point, given a path. As we can see, the resulting momentum(and force) at the destination is dependent on the path chosen and the particular geometry of the particular spacetime, i.e whether you are in a stationary or rotating frame of reference. So forces at a distance, as described by Mach's Principle, cannot really obey Newton's Third Law under local symmetry.
Under closer analysis, Newton's third law is really a meta-law and is fundamentally equivalent to conservation of momentum. This is a consequence of Noether's Theorem which states that any change of the equations of motion under an explicit symmetry must result in a conservation law.
Physicists and mathematicians define a “symmetry” as a coordinate transformation that can be done to a system that leaves its essential features unchanged.
A circle has a lot of symmetry, as we can rotate it around the middle by any angle, and after the rotation it remains the same circle. We can also reflect it around an axis down the middle. A square, by contrast, has some symmetry, but less — we can reflect it around the middle, or rotate by some number of 90-degree angles, but if we rotated it by an angle that wasn't a multiple of 90 degrees we wouldn't get the same square back. A random scribble doesn't have any symmetry at all; anything we do to it will change its appearance.
More exactly, Noether’s theorem says that if you can continuously change some coordinate variable 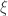 without changing the environment, then there is a conserved quantity
without changing the environment, then there is a conserved quantity  equal to
equal to
where 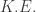 is the kinetic energy and
is the kinetic energy and  is the time rate of change of
is the time rate of change of  . As an example, the kinetic energy can be written
. As an example, the kinetic energy can be written 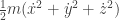 , so that if the system is unchanged by translations along the
, so that if the system is unchanged by translations along the  direction, then the conserved quantity is
direction, then the conserved quantity is  , which is the momentum in the
, which is the momentum in the  direction.
direction.
Noether’s Theorem also allows you to identify less obvious conserved quantities. For example, imagine that the force-emitting object is a cylinder with a helical coil wrapped around it, like this: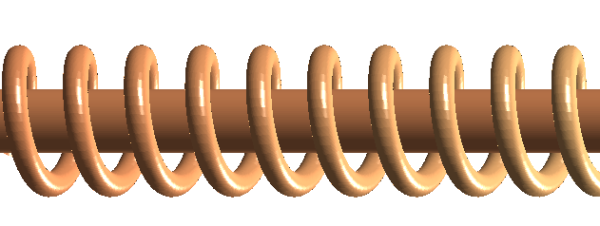

This environment is no longer unchanged by small translations in the 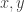 or
or  directions, nor by small rotations around any of the axes. It is, however, unchanged by a particular combination of translation and rotation. Specifically, if
directions, nor by small rotations around any of the axes. It is, however, unchanged by a particular combination of translation and rotation. Specifically, if  is the distance between coils of the helix then the environment is unchanged when you simultaneously rotate 360 degrees around the
is the distance between coils of the helix then the environment is unchanged when you simultaneously rotate 360 degrees around the  axis and translate by
axis and translate by  in the
in the  direction. Any small translation/ rotation done in that same proportion also leaves the environment unchanged. Noether’s Theorem therefore guarantees that a particular combination of linear momentum and angular momentum will be conserved forever.
direction. Any small translation/ rotation done in that same proportion also leaves the environment unchanged. Noether’s Theorem therefore guarantees that a particular combination of linear momentum and angular momentum will be conserved forever.
Specifically, you can work out from the equation above that
Energy conservation appears naturally from Noether’s Theorem when you assume that the environment is symmetric with respect to translations in time. Momentum conservation appears in environments which are symmetric in space.
In all cases, the violation of Newton's 3rd law in a local symmetry is a result of conservation of momentum.
In General Relativity the gravitational "field" is really an illusion in a sense, an illusion created by how mass-energy density warps spacetime and how gravitational fields propogate in advanced disturbances creating the effect of the inertial reaction force. We might be convinced then that the issue of "action at a distance" to describe the effect of Ernst Mach is eliminated.
In this sense the fields described in General Relativity are just book-keeping devices for the delayed interaction of sources.
To contrast, in Quantum Field theory, the field itself is considered a physical quantity and carries with it an energy density. In Quantum field theory, excitations in the field are in effect quantised in a form of angular momentum in a rotationally symmetric field space, thereby eliminating the standing notion that there is something particularly different between the nature of the field (electromagnetic, strong, weak) and interactions within it.
Variables such as spin, charge, quark color and so forth are quanta of the field in this view.
General Relativity however still views the nature of the gravitational field as a book keeping device for delayed interaction of sources as it still sees the field itself as carrying an energy density with no explanation as to how the field quanta influences particles.
General relativity was emphasized by the geometrical picture of gravity. Fundamentally however, General relativity must itself be some form of lower order theory which arises from a theory of gravitation from spin-2 particles of mass-0, i.e. gravitons.
The initial form of general relativity was made to be as simple as possible, which is a result of Einstein himself using only 2d-order Partial Differential Equations (PDEs), rejecting the higher order (3rd, 4th, ect) PDEs.
An Effective Quantum Field Theory for gravity would not only include the higher terms but would also include coefficients on the geodesic length which would be on the order of Planck's Constant.
This would mean that most of the higher order effects would only be seen at the order of the Planck Length, i.e. at the Big Bang or inside a Black Hole.
Moreover, the very nature of General Relativity always generates singularities when you do any calculations in modelling how it moves small amounts of matter, such as atoms or electrons, using the Path Integral which is the most fundamental comprehension of how objects move.
In effect, the higher order effects of an Effective Field Theory of gravity has to be modeled independently of the lower order effects to make sensible predictions of its interaction with elementary particles. Something which still lies beyond the scope of current theoretical physics.
Variables such as spin, charge, quark color and so forth are quanta of the field in this view.
General Relativity however still views the nature of the gravitational field as a book keeping device for delayed interaction of sources as it still sees the field itself as carrying an energy density with no explanation as to how the field quanta influences particles.
General relativity was emphasized by the geometrical picture of gravity. Fundamentally however, General relativity must itself be some form of lower order theory which arises from a theory of gravitation from spin-2 particles of mass-0, i.e. gravitons.
The initial form of general relativity was made to be as simple as possible, which is a result of Einstein himself using only 2d-order Partial Differential Equations (PDEs), rejecting the higher order (3rd, 4th, ect) PDEs.
An Effective Quantum Field Theory for gravity would not only include the higher terms but would also include coefficients on the geodesic length which would be on the order of Planck's Constant.
This would mean that most of the higher order effects would only be seen at the order of the Planck Length, i.e. at the Big Bang or inside a Black Hole.
Moreover, the very nature of General Relativity always generates singularities when you do any calculations in modelling how it moves small amounts of matter, such as atoms or electrons, using the Path Integral which is the most fundamental comprehension of how objects move.
In effect, the higher order effects of an Effective Field Theory of gravity has to be modeled independently of the lower order effects to make sensible predictions of its interaction with elementary particles. Something which still lies beyond the scope of current theoretical physics.
Studies in the field of quantum gravity have led to the creation of different descriptions and models of spacetime metrics. The creation of different metrics to expand General Relativity can sometimes create theoretical models that radically changes our concept of motion.
The most famous metric that models exotic motion in spacetime is Alcubierre's metric, so it can be used as the archetypal probe into how to model exotic motion in spacetime.
Alcubierre Metric: The Idea of a Stable Warp Field
In 1994, Miguel Alcubierre developed a geodesic equation to describe space-time warped in a bubble around a ship, creating a "warp drive".
The warp drive proposed by Alcubierre could achieve near light speeds and even faster-than-light speeds by distorting space-time. To accomplish this, a theoretical device would generate a field of negative energy that would squeeze or stretch space-time, creating the bubble. The bubble would ride the distortions like a surfer on a wave.
As evidenced by cosmic inflation in the big bang, in certain conditions space-time can expand so quickly that objects can move faster than the speed of light.
The theoretical basis for the operation of this experiment is that a massive object causes spacetime to curve and in-turn spacetime tells a massive object how to move and accelerate.
It is postulated that spacetime curvature can be modified using powerful electromagnetic fields to reduce the inertial mass of a starship.
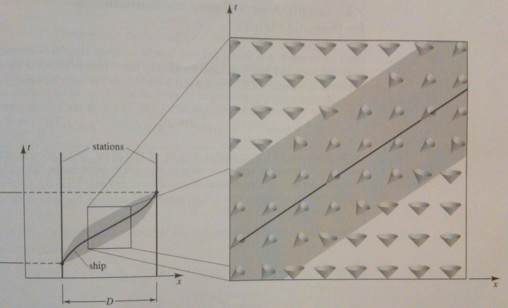
By bending spacetime in a particular way you can make it so that locally you move slower than light, but that the overall effect is faster-than-light travel. The arrangement of matter and energy that allows for this is unfortunately impossible. This diagram is from page 145 of “Gravity”, by Hartle.
In the weakest implementation of this theory a starship can be made to accelerate as if the inertial mass of the starship were reduced making near light speed possible using simple electric thrusters. However, in the most advanced implementation of this experiment when the energy of the electromagnetic fields cause the inertial mass of a starship to become imaginary the starship in the warp bubble will become tachyonic and will be capable of moving faster than the speed of light. In its advanced form the object in the warp bubble is isolated from the rest of the universe allowing the warp bubble to become a local frame of reference where Faster Than Light (FTL) travel does not violate the local speed of light (c).
A Tachyon is a hypothetical particle postulated to move at a velocity greater than the speed of electromagnetic radiation, such that as the particle accelerates it loses energy. Of the two properties rest mass and energy, one must be real and the other imaginary. If a tachyon exists it may be detected through the emission of Cerenkov radiation (a kind of electromagnetic shock wave) in a particle accelerator or by cosmic ray collisions.
The Alcubierre metric was the first attempt to design a theoretical model that can make some predictions about what is necessary for a warp drive.
Here also, is some plots showing some of the key aspects of the model including the Alcubierre Metric, Light Cone and Energy density around a hypothetical warp ship.
Miguel Alcubierre's famous warp metric is of the form:
f(rs) = -----------------------------------------
2tanh(sR)
Using such a metric, generated around a ship, we can picture a craft surfing what is essentially a gravitational wave front:
(1) The vertical dimension represents how much a given volume of space-time expands or contracts in Alcubierre’s model. Positive values [red] imply an expansion in space-time caused by negative mass-energy density in Einstein's theory of General Relativity. When space-time expands behind a craft, it propels the ship forward.
(2) Inside the warp bubble, neutral space-time would leave the ship undisturbed. Passengers would experience a zero-G environment. Artificial gravity can be created in a portion of the ship using rotation to create a stable centrifugal force.
(3) Negative values [blue] imply a contraction in space-time caused by positive (i.e."normal") mass-energy density. The contraction balances the expansion of space-time as the bubble moves forward. Combined this allows the ship to "surf" the gravitational wave front.
These plots were developed using a Matlab code I wrote which is available for copying at the end of the page.
In fact what Alcubierre proposed as a "warp drive" is using a form of bipolar (or "dual") gravitational waves as a method of propulsion. Gravitational waves in general relativity are planar and hence each wave expands and contracts, however, the Alcubierre metric in principle suggest that such an effect could be bipolar, possibly explaining the necessity for a "negative mass-energy density" requirement.
What this metric truly suggest is that such a manipulation of space would cause spacetime to propel a localized region of space (refereed to as a warp bubble) by expanding and contracting the metric field. Since gravitational radiation is believed to propagate at the speed of light the prolusion of this space is similar in principle as to how electric and magnetic field cause electromagnetic radiation to propagate.
This however only effects the external properties of the warp bubble while internally the effects of the bubble could be as large as one wished (this deals with the construction of energy densites within the field). The main benefit of this theory is that it dramatically lowers the negative energy requirements, thereby making warp drive look feasible with an advanced technology.
Nevertheless, such a metric requires a mass-energy density the size of Jupiter concentrated in a region around a football field. Engineering specifications of such a device is therefore literally in outer space and no sane person would, or should, fund it.
Ideas about how such a device would work are, however, free. Theoretical physics is the cheapest form of science to fund, even cheaper than mathematics. Unlike mathematics where the mathematician only needs a pencil, paper and a waste paper bin for the ideas he throws out the theoretical physicist can be kept happy without the waste paper bin!
As Richard Feynman once joked in his lecture series on the "Character of Physical Law" "Every theoretical physicist has at least 6 different competing theories for the same phenomena floating around in his head, each describing the same phenomena in a different way but coming to similar conclusions"
However, as with any theory, there are loopholes around such scenarios as faster than light travel which lead to paradoxes. One such paradox is that if a ship is travelling faster than c, then should'nt it appear to be travelling backwards in time? Paradoxes should be therefore expected as we do not fully understand the true nature of gravitational interactions particularly with atomic or subatomic phenomena.
Physical Principles needed to "Build" Alcubierre's Warp Drive
The warp drive proposed by Alcubierre could achieve near light speeds and even faster-than-light speeds by distorting space-time. To accomplish this, a theoretical device would generate a field of negative energy that would squeeze or stretch space-time, creating the bubble. The bubble would ride the distortions like a surfer on a wave.
As evidenced by the uniformity of the Cosmic Microwave Background from the Big Bang, which is explained by inflationary cosmology, space-time can expand so quickly that objects can move faster than the speed of light.
Therefore the current models of physics generally allow for the existence of a warp field that can accelerate objects faster than the speed of light.
The real questions to ask is whether or not such a warp field can exist on macroscopic scales and if so can it remain stable for long enough to observe its effects, on light in a laser interferometer for example.
Moreover it is unknown how it is technologically possible, i.e under what conditions does matter allow for the creation of a negative energy density?
In 1948, Theoretical Physicists Hendrik Casimir and Dirk Polder proposed that a negative presssure can exist due to quantum vacuum fluctuations operating on very small scales in space and time and that if two uncharged metallic plates in a vacuum, placed a few micrometers apart the quantum fluctuations should create a force between the 2 plates due to a differential vacuum energy density between the inside and outside of the plates.
Classical Experimental Setup of the Casimir Effect
In a classical description, the lack of an external field automatically means that there is no field between the plates, and no force would be measured between them. However when the zero-point field is instead studied using the QED vacuum of quantum electrodynamics, it is seen that the plates do affect the virtual photons which constitute the field, and generate a net force.
The force can give either an attraction or a repulsion depending on the specific arrangement of the two plates.
Although the Casimir effect can be expressed in terms of virtual particles interacting with the objects, it is best described and more easily calculated in terms of the zero-point energy of a quantized field in the intervening space between the objects.
It was not until 1997, however, that a direct experiment, by Steve Lamoreaux, quantitatively measured the force (to within 15% of the value predicted by the theory)
Previous work in the 1970's had observed the force qualitatively, and indirect validation of the predicted Casimir energy had been made by measuring the thickness of liquid helium films by Sabisky and Anderson in 1972. Subsequent experiments with liquid Helium-3 approach an accuracy of a few percent.
Using Bose-Einstein Condensates it may also be possible to suppress background effects occurring between individual molecules, such as the Van Der Waals Forces, which will help to quantify the necessary boundary conditions in the second quantisation calculations of Quantum Electrodynamics. This would allow the effect of the vacuum to become dominant in the medium and allow for a more direct observation of negative energy density affecting it.
This could also allow for further studies in solid state physics on how the Casimir effect could be controlled at the nanoscale and what physics and applications can be gained from it.
Some of this research, although abstract, may help us to understand how electronic transitions occur at the smallest of scales and how to suppress noise, such as that caused by the Casimir effect, in nanoscale circuitry, such as in the emergent fields of quantum circuitry.
Therefore by probing some deep questions of physics, and examining theories such as "Warp Drives" we may uncover a great deal of knowledge and gain proposals for some interesting experiments, perhaps even stumbling upon the foundations of warp drive itself along the way.
Some final thoughts...
The fact that we can even ask some of these questions concerning negative energy fluctuations, higher dimensions and the controlled warping of spacetime is a testament to how advanced topics in science can capture the imagination and motivate us to look beyond parochial assumptions. Such thinking is healthy for the imagination and helps us realize that we have some potential to achieve great powers through the use of our intelligence and imagination.
The physics of "Warp Drive" is complicated, to say the very least, and may be considered far-fetched by today's standards, perhaps more far-fetched than flying carpets are to supersonic aircraft.
One question that could be asked is "how can humans even begin to manipulate space and time?". More understandable of course is the response that "this sounds like science fiction". However, we should not let that statement deter us from asking questions. Although nature has not thrown warp fields at us the same way it has thrown lightning, earthquakes and starlight at us does not mean that they are impossible to create.
To put this into perspective, consider again the analogy of magnetism. The interstellar magnetic field is about a nanoTesla, or about one-fifty thousandth of the Earth's field, which ranges from about 25 to 65 microTeslas at the surface. This is staggeringly small, as is Jupiter's magnetic field is about 10 times stronger than Earth's. Even our Sun's field, though extensive is nothing particularly alarming with sunspot's, where the field lines reach their most intense activity, reaching 0.5 Teslas at most.
If this is all we knew about magnetism, harnessing magnetism for any practical purpose would seem unlikely and achieving field strength's to rival the stars would seem far-fetched and fictitious.

However, a tiny Neodymium magnet you can hold in your hand exhibits magnetic fields of 1.3 Teslas, 100 million times stronger than the interstellar field and almost 3 times as large as sunspot fields.
Therefore even on the stellar scale humans can best nature at some things. Although manipulation of gravity might seem beyond conventional engineering the fact that the idea can be discussed in the context of General Relativity fairly easily will no doubt continue to allow it to be used as an interesting introduction to some of the physics and mathematics used in General Relativity in general, which already has applications and presence in astronomy and space science today.
Matlab Code for Alcubierre Warp Drive Model:
[x, y] = meshgrid([-10:.1:10],[-10:.1:10]);
%Radius R
R=3;
sigma = 1;
%Ship's Position
xs = 0;
for i=1:length(x);
for j=1:length(x);
%Alcubierre Warp Metric
z(i,j)=-1*(tanh (sigma*sqrt(abs(x(i,j)^2+y(i,j)^2-16))+R)-tanh(sigma*sqrt(abs(x(i,j)^2+y(i,j)^2-16))-R))...
*tanh(sigma*R)*x(i,j);
%invariant length of space
rs(i,j) = sqrt(abs(x(i,j)^2 + y(i,j)^2 + z(i,j) )) ;
%Energy density function
E(i,j) = (tanh(sigma*(rs(i,j) + R)) - tanh(sigma*(rs(i,j) - R)))/(tanh(sigma*R)) ;
end
end
%Light Cone
figure(1)
mesh(x,y,rs)
axis([-10 10 -10 10 -10 10 -10 10])
view([158,26])
colormap(jet)
%Energy Density
figure(2)
mesh(x,y,E)
axis([-10 10 -10 10 -10 10 -10 10])
view([158,26])
colormap(jet)
%Alcubierre Warp Metric
figure(3)
mesh(x,y,z)
axis([-10 10 -10 10 -10 10 -10 10])
view([158,26])
colormap(jet)








